Inverse Functions And Logarithms 3

Inverse Trigonometric Functions

We know that the sine function y=sin x is not one-to-one (use the Horizontal Line Test). But the function f(x)=sin x,  is one-to-one so we can find the inverse of it

\[{\sin ^{ - 1}}y = x \Leftrightarrow y = \sin x,\frac{{ - \pi }}{2} \leqslant x \leqslant \frac{\pi }{2}\]

\[Thus, - 1 \leqslant y \leqslant 1\]

\[Note:{\sin ^{ - 1}}x \ne \frac{1}{{\sin x}}\]

Remember:

So now we know that the range of sine is between -1 and 1 

and the range of inverse sine is between 

for that, we should know the facts that

1. \[{\sin ^{ - 1}}(\sin x) = x,\,for\,\frac{{ - \pi }}{2} \leqslant x \leqslant \frac{\pi }{2}\]
2. \[\sin ({\sin ^{ - 1}}x) = x,\,for\, - 1 \leqslant x \leqslant 1\]

the same thing we can do with cos and tan 

\[{\cos ^{ - 1}}(\cos x) = x,\,for\,0 \leqslant x \leqslant \pi \]

\[\cos ({\cos ^{ - 1}}x) = x,\,for\, - 1 \leqslant x \leqslant 1\]

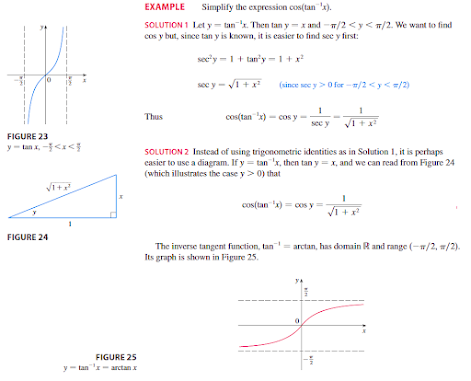
\[{\tan ^{ - 1}}x = y \Leftrightarrow \tan y = x\,\,,\,and\,\,\frac{{ - \pi }}{2} \leqslant y \leqslant \frac{\pi }{2}\]